Nuclear medicine can be defined as the practice of making patients radioactive for diagnostic and therapeutic purposes. The radioactivity is injected intravenously, rebreathed, or ingested. It is the internal circulation of radioactive material that distinguishes nuclear medicine from diagnostic radiology and radiation oncology in most of its forms. This section will examine only the diagnostic use and will concentrate on methods for detecting the radioactivity from outside the body without trauma to the patient. Diagnostic nuclear medicine is successful for two main reasons It can rely on the use of very small amounts of materials (picomolar concentrations in chemical terms) thus usually not having any effect on the processes being studied, and the radionuclides being used can penetrate tissue and be detected outside the patient. Thus the materials can trace processes or “opacify” organs without affecting their function.
Parameters for Choices in Nuclear Medicine
Of the various kinds of emanations from radioactive materials, photons alone have a range in tissue great enough to escape so that they can be detected externally. Electrons or beta-minus particles of high energy can create bremsstrahlung in interactions with tissue, but the radiation emanates from the site of the interaction, not the site of the beta ray’s production. Positrons or beta-plus particles annihilate with electrons to create gamma rays so that they can be detected. For certain radionuclides, the emanation being detected is x-rays, in the 50- to 100-keV energy range.
The half-lives of materials in use in nuclear medicine range from a few minutes to weeks. The half-life must be chosen with two major points in mind: the time course of the process being studied and the radiation dose to the target organ, that is, that organ with the highest concentration over the longest time (the cumulated activity or area underneath the activity versus time curve). In general, it is desired to stay under 5 rad to the target organ.
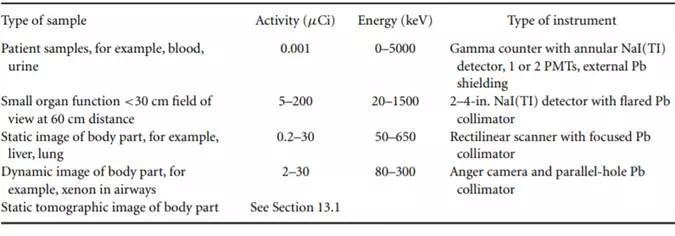
The choice of the best energy range to use is also based on two major criteria: the energy that will penetrate tissue but can be channeled by heavy metal shielding and collimation and that which will interact in the detector to produce a pulse. Thus the ideal energy is dependent on the detector being used and the kind of examination being performed. Table 13.1 describes the kinds of gamma-ray detection, the activity and energy ranges, and an example of the kind of information to be gained. The lesser amounts of activity are used in situations of lesser spatial resolution and of greater sensitivity. Positron imaging is omitted because it is treated elsewhere.
Radiation dose is affected by all the emanations of a radionuclide, not just the desirable ones, thus constricting the choice of nuclide further. There can be no alpha radiation used in diagnosis; the use of materials with primary beta radiation should be avoided because the beta radiation confers a radiation dose without adding to the information being gained. For imaging, in addition, even if there is a primary gamma ray in the correct energy window for the detector, there should be no large amount of radiation, either of primary radiation of higher energy, because it interferes with the image collimation, or of secondary radiation of a very similar energy, because it interferes with the perception of the primary radiation emanating from the site of interest.
For imaging using heavy-metal collimation, the energy range is constrained to be that which will emanate from the human body and which the collimation can contain, or about 50 to 500 keV.
Detectors must be made from materials that exhibit some detectable change when ionizing radiation is absorbed and that are of a high enough atomic number and density to make possible stopping large percentages of those gamma rays emanating (high sensitivity). In addition, because the primary gamma rays are not the only rays emanating from the source—ahuman body and therefore a distributed source accompanied by an absorber — there must be energy discrimination in the instrument to prevent the formation of an image of the scattered radiation. To achieve pulse size proportional to energy, and therefore to achieve identification of the energy and source of the energy, the detector must be a proportional detector. This means that Geiger-Muller detection, operating in an all-or-none fashion, is not acceptable.
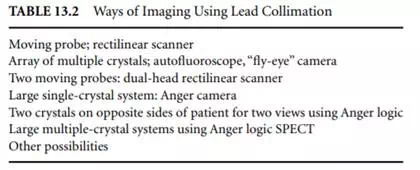
Gaseous detectors are not practical because their density is not great enough. Liquid detectors (in which any component is liquid) are not practical because the liquid can spill when the detector is positioned; this problem can be compensated for if absolutely necessary, but it is better to consider it from the outset. Another property of a good detector is its ability to detect large numbers of gamma rays per time unit. With detection capabilities to separate 100,000 counts per second or a dead time of 2 msec, the system is still only detecting perhaps 1,000 counts per square centimeter per second over a 10 × 10 cm area. The precision of the information is governed by Poisson statistics, so the imprecision in information collected for 1 sec in a square centimeter is ±3% at the 1 standard deviation level. Since we would hope for better spatial resolution than 1 cm2, the precision is obviously worse than this. This points to the need for fast detectors, in addition to the aforementioned sensitivity. The more detector that surrounds the patient, the more sensitive the system will be. Table 13.2 lists in order from least sensitive to most sensitive some of the geometries used for imaging in nuclear medicine. This generally is also a listing from the older methods to the more recent.
For the purposes of this section, we shall consider that the problems of counting patient and other samples and of detecting the time course of activity changes in extended areas with probes are not our topic and confine ourselves to the attempts made to image distributions of gamma-emitting radionuclides in patients and research subjects. The previous section treats the three-dimensional imaging of these distributions; this section will treat detection of the distribution in a planar fashion or the image of the projection of the distribution onto a planar detector.
