The fundamental image characteristics of resolution and field of view (FOV) are completely determined by the characteristics of the k-space scan. The extent of the coverage of k-space determines the resolution of the reconstructed image. The resolution is inversely proportional to the highest spatial frequency acquired:
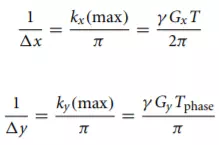
where Gx is the readout gradient amplitude and T is the readout duration. The time Tphase is the duration of the phase-encode gradient Gy . For proton imaging on a 1.5-T imaging system, a typical gradient strength is Gx = 1 G/cm. The signal is usually read for about 8 msec. For water protons, γ = 26, 751 rad/sec/G, so the maximum excursion in kx is about 21 rad/mm. Hence we cannot resolve an object smaller than 0.3 mm in width. From this one might be tempted to improve the resolution dramatically using very strong gradients or very long readouts. But there are severe practical obstacles, since higher resolution increases the scan time and also degrades the image SNR.
In the phase-encode direction, the k-space data are sampled discretely. This discrete sampling in k-space introduces replication in the image domain. If the sampling in k-space is finer than 1/FOV, then the image of the object will not fold back on itself. When the k-space sampling is coarser than 1/FOV, the image of the object does fold back over itself. This is termed aliasing. Aliasing is prevented in the readout direction by the sampling filter.
